Microrheology: Running measurements on the Zetasizer ZSP/ZS

1.1 DLS microrheology technique

DLS microrheology is based around extracting linear rheological properties from the motion of colloidal probe (tracer) particles, of known size, undergoing thermal fluctuations (from Brownian motion) in a system at thermodynamic equilibrium.

Relationships have been derived that enable quantitative rheological data over a wide range of frequencies, and which have been shown to hold, in general, across a variety of complex fluid types.

Data can be obtained for the frequency-dependent viscoelastic moduli - G', elastic (storage) modulus and G", viscous (loss) modulus - from which the frequency-dependent complex viscosity, η*, can also be calculated.

Since no external forces are exerted on the probe (tracer) particles, DLS microrheology is a passive technique, and can also be described as thermal diffusion microrheology.

DLS microrheology measurements are particularly suited to the measurement of low viscosity and weakly-structured materials, such as polymer and biopolymer (e.g. protein) solutions. The technique extends rheological data into the high frequency range, which is a requirement to access the short timescale dynamics of such systems (and goes well beyond the inertia-limited capabilities of mechanical rheometers in this respect).

1.2 Microrheology application

A microrheology test requires the measurement of the correlation function of the probe (tracer) particles in the sample under test. The Mean Square Displacement (MSD) of probe particles is then calculated, from which the rheological properties of the sample are subsequently evaluated (using the Generalized Stokes-Einstein Relation).

Note that in order to obtain reliable microrheology data, there are certain conditions for the tracer particle-sample matrix combination that must be met:

• Interactions between the tracer particles and the sample matrix should be minimal
• The concentration of probe particles should be such that the scattering signal is dominated by the probe particle scattering, as compared to the scattering from the sample matrix (whilst maintaining the single scattering condition)
• The probe particles should be well dispersed in the sample
• The probe particle size should be larger than the relevant microstructural length scale e.g. mesh size in a polymer network, such that it probes the bulk material response i.e. the assumption of continuum viscoelasticity holds.

The use of a defined experimental procedure is recommended in order to ensure a robust method is generated for DLS microrheology characterization of a particular complex fluid type.  Therefore, the microrheology functionality in the Zetasizer software includes 'pre-measurement' steps to allow evaluation of the above conditions.

The 'pre-measurement' steps within the microrheology application are as follows:

1. Optimization measurement to assess tracer particle-sample matrix interactions (using zeta potential test protocols)
2. Optimization measurement to assess tracer particle scattering relative to sample matrix scattering (using size test protocols) and predict required tracer concentration
3. Microrheology measurement to determine the auto-correlation function for the sample-tracer dispersion and extract rheological parameters

This methodology is aimed at ensuring the important conditions for a particular tracer particle-sample matrix combination are met.

Note that once a particular sample type has been assessed for a suitable probe particle type, size and concentration, then only an autocorrelation function (ACF) measurement needs to be run to extract microrheology data.

At all measurement steps within the microrheology application, user guidance is provided in the software. Expert Advice on data quality is also provided.

1.2.1    Optimization - Tracer Compatibility: Assess tracer particle-sample matrix interactions using zeta potential test

Measurement step 1 in the microrheology application is used to assess the suitability of probe particle chemistry to minimize particle-matrix interactions. This is done by measuring:

1. The zeta potential of the tracer particles in the dispersant (solvent) being used for the microrheology test

2. The zeta potential of the tracer particles in the dispersant AND sample being used for the microrheology test

The concentration of probe particles used will depend to a great extent on the concentration of the sample in the dispersant (solvent). For the initial measurement of the zeta potential (tracer/solvent only and tracer/sample), the following concentrations are suggested starting points:

• Tracer particles in dispersant - 5 µL neat tracer particle suspension to 10 mL dispersant (solvent) (0.5 µL/mL)
• Tracer particles in presence of sample - Add one drop of the dispersed (diluted) sample (approx. 0.05 mL) to approximately 5 mL of the previously prepared tracer dispersion allowing sufficient mixing and equilibration time prior to measurement

Note on filtering of tracer particle suspensions for use in microrheology testing:

For the most reproducible data, it is recommended that the tracer particle suspension is filtered with an appropriately sized filter (i.e. filter size larger than the tracer size)

The tracer optimization step requires the use of a zeta potential capable cell - there are standard SOPs in the software for:

• ZEN1002 Universal dip cell
• DTS1070 Disposable folded capillary cell

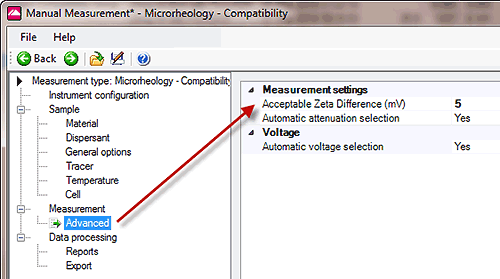
The microrheology software compares the two measured zeta potential results and tests to see if sample measurement is within a set tolerance of the tracer measurement (the 'Acceptable Zeta Ratio'). The software default value for this difference is 5 mV, which can be changed in the advanced settings - Figure 2.

Figure 1: Changing the Acceptable Zeta Ratio (mV) in the software

The zeta potential of a colloidal particle will change if the surface properties of the particle change e.g. if components of the dispersant matrix, such polymer or protein molecules, adsorb onto the particle surface.

For a DLS microrheology measurement - if the zeta potential of the probes in the presence of the sample is significantly different from the value obtained in the dispersant (solvent), this may indicate strong particle-matrix interactions. Such interactions will inevitably affect the rheological data obtained.

Malvern can offer a limited number of surface chemistries for microrheology tracer particles although other surface chemistries can be made available upon request. The different surface chemistries can be used to test the level of interaction between sample and tracer (using zeta potential measurements as above), such that an optimum tracer particle surface chemistry can be selected for the particular sample type under test.

It is important to note that interactions between the sample and the tracer particles may not be immediately apparent as they can take some time to manifest themselves. Equally, if the sample is not optimally dispersed prior to measurement then the measurement will not be truly representative of the sample. The use of gentle mixing (for example using a sample roller) will help to disperse the tracer particles if required.

An alternative or complimentary approach for evaluating tracer-sample interaction is to measure the rheological behaviour of the sample with and without tracer present on a rotational rheometer. If no interaction is occurring then the rheological response (specifically G') should be similar between the two samples, while a significant difference would suggest interaction. For users with instruments that do not have a zeta capable instrument but have access t o a rheometer this may be the only option for evaluating interaction.

1.2.1.1. Guidelines if zeta potential comparison is outside the 'Acceptable Zeta Difference'

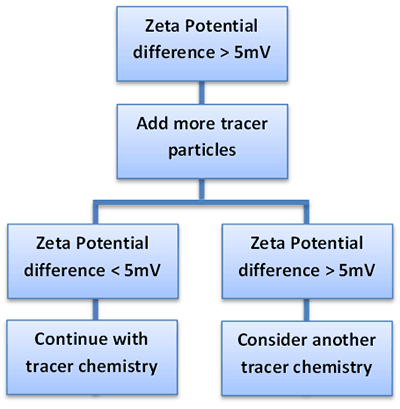
There are two possible causes if the measured zeta potential shows significant differences between the dispersant/tracer and the sample/tracer systems:

1. The tracer particle concentration is not high enough in the sample
   If the tracer concentration is too low, the zeta potential result will be dominated by the sample, not the tracer. Adding more tracer particles and re-testing can show whether this difference is due to concentration rather than interaction with the sample.
2. The tracer particles and sample are interacting
   If the tracer and sample are interacting, adding more tracer particles will not improve the difference in zeta potential (unless so much is added that it completely dominates the zeta measurement). This result may be indicative of significant tracer particle-sample matrix interactions, and different probe chemistry should be investigated.

The decision making process for choosing an appropriate tracer particle surface chemistry is shown in Figure 2:

Figure 2: Decision making process for probe surface chemistry

1.2.2 Optimization - Tracer Concentration: Predict optimum tracer concentration by measuring scattering relative to sample matrix

The second measurement option in the microrheology application is used to assess the level of tracer particle scattering against sample matrix scattering, and use this ratio to predict the tracer concentration required to give dominant scattering.

The following guidance is for the suggested concentration of tracer particles to be added to the sample:

• Add approximately 5 µL of neat tracer particle suspension to 1 mL of sample (or a relative amount depending on the type of cell being used) and ensure the particles are well dispersed. The exact concentration should then be entered in the tracer properties window as shown in Figure 3 .

Figure 3 - Changing the tracer concentration in a concentration optimisation step

Once prepared the sample should be transferred to a size capable measurement cell and the measurement started. The measurement is effectively just a size measurement and measures the intensity of the tracer particles at the inputted concentration relative to that of the sample. This relative value is then used to calculate the tracer concentration required to give a relative intensity ratio of 95% (19:1), which is the minimum recommended to ensure the tracer scattering will dominate the measurement. This calculation is made on the basis that for single scattering there should be a linear correlation between concentration and intensity.

This value is displayed in a prompt at the end of the test but also recorded in the results summary table.

Note on filtering of tracer particle suspensions for use in microrheology testing:

For the most reproducible data, it is recommended that the tracer particle suspension is filtered with an appropriately sized filter.

Note that conditions of single scattering are required for DLS microrheology - if the concentration of tracer particles in the sample is too high, the system will become highly turbid. It is therefore important to use a concentration of tracer particles that is just sufficient to mask the sample scattering, and not put in so much that the sample becomes highly turbid (leading to multiple scattering).

1.2.3 Microrheology measurement

A microrheology measurement:

• Records the correlation function of the tracer particles in the sample
• Evaluates the Mean Square Displacement (MSD) of the tracer particles
• Calculates the rheological properties of the sample using the Generalized Stokes-Einstein Relation

The optimization steps for Tracer Compatibility and Tracer Concentration are optional but are useful tools for assessing whether the tracer chemistry is likely to be suitable for a particular sample and if so what is the minimum concentration that can be used. If this information is already known then the user can proceed to the microrheology measurement.

The only extra information the user requires for the microrheology test is the hydrodynamic size of the tracer particle in the sample solvent or buffer. This step is not included as an optimization step in the microrheology application as it just requires running a standard size measurement of the tracer in the solvent, which can be done through a standard size measurement.

There are two important points to note here:

1. The tracer particle size should be measured to make the microrheology result as accurate as possible. The size of the tracer particle may change slightly from the nominal size, depending on the pH of the dispersant or presence of electrolyte. This is because the electrical double layer (Debye layer) will change depending on the ionic strength of the dispersant, thus affecting the measured hydrodynamic size of the particle.
2. The tracer size must be significantly larger than the measured size of the sample.

Under these conditions, the tracer particles probe the bulk material response i.e. the assumption of continuum viscoelasticity holds. If a tracer particle size is small on the microstructural length scale of the sample matrix, then bulk rheological properties will not be recovered from the microrheology measurement.

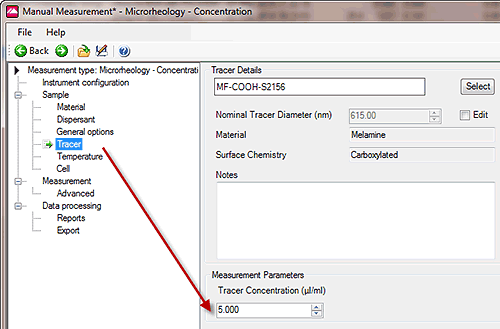
Some tracer particles are already set-up in the software under Tracer Details. These include tracer particles supplied by Malvern and can be 'added to' and edited by the user as required. For Malvern supplied particles the Nominal Tracer Size is usually the size on the bottle which may differ from the hydrodynamic size in the sample solvent. This value can be changed by ticking the Edit box. It will be this value which is used in the microrheology calculation. The tracer window is shown in Figure 4 .

Figure 4: Editing the tracer properties in a microrheology measurement

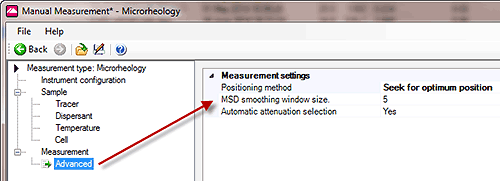
The algorithm used to convert the measured Mean Square Displacement to rheological data requires an accurate estimate of the local gradient at each discrete measurement (or delay) time. To improve the accuracy and quality of this conversion an optional smoothing window can be applied to the the Mean Square Displacement vs Time curve as shown in Figure 7. The default window size is 5 points but this can be edited after the test if required, as shown in Figure 5.

Figure 5: Applying smoothing in a microrheology measurement

1.4 Microrheology Charts and Results

The following charts are included in the microrheology workspace:

1.4.1 Correlogram

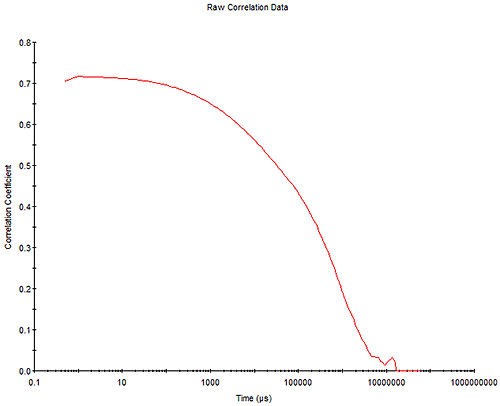
The measured correlation function of the tracer particles in the sample as shown in Figure 6.

• The Correlation Function shows how the fluctuation in scattering intensity from the tracer particles varies with delay time.

This is usually a good measure of data quality with a smooth correlation function and intercept tending to unity preferred. It should be noted, however, that the intercept is also related to the extent of elasticity in the system (degree of relaxation) so more elastic systems will tend to have lower intercepts.

Figure 6: Correlation function vs time

1.4.2 Mean Square Displacement (MSD)

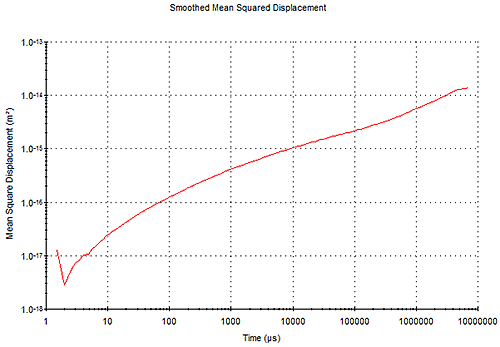
The Mean Square Displacement (MSD) is derived from the correlation function, and is a representation of the time dependent movement of the tracer particles within the sample as shown in Figure 7.

• A linear response on log-log scale indicates purely diffusive motion of the tracer particles - as would occur in an ideal Newtonian liquid
• Deviations from linearity with time of the MSD of the tracer particles is indicative of sample viscoelasticity (and sub-diffusive motion of the tracer particles)

Figure 7: Mean Square Displacement (MSD) vs time

1.4.3 Shear Modulus (G', G")

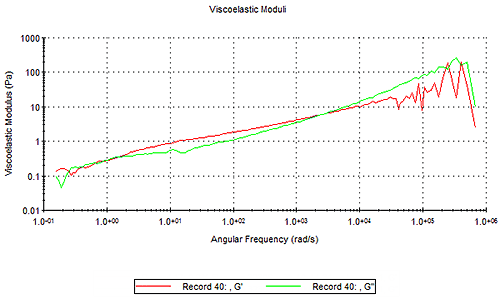
The Moduli tab shows the viscoelastic moduli versus frequency for the sample under test - two sets of data are shown on the chart as shown in Figure 8

• G', elastic (storage) modulus
• G", viscous (loss) modulus

The viscous modulus (G") will be dominant at lower frequencies (i.e. longer timescales) and the elastic modulus (G') will be dominant at higher frequencies (i.e. shorter timescales). Where a cross-over between the G' and G" occurs, the frequency of the crossover relates to an important relaxation time of the material. For low viscosity, non-Newtonian materials, the cross-over point is often at a very high frequency, which is inaccessible from inertia-limited mechanical rheometry techniques, but these dynamics can be probed by the extended frequency range of DLS microrheology.

For the types of sample applicable to DLS microrheology - low viscosity and weakly-structured materials such as polymer and biopolymer (protein) solutions - it is reasonable to expect the viscous (loss) modulus G" will dominate for at least most of the measured frequency range (and indeed for very weakly structured materials, the G' component may be very noisy).

Figure 8: Viscoelastic moduli (G' and G") versus angular frequency

1.4.4 Complex viscosity (η*)

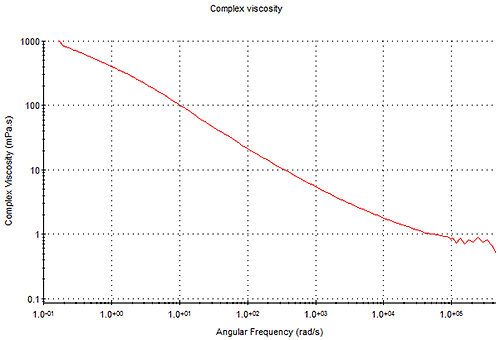
The frequency-dependent complex viscosity, η*, as shown in Figure 9 , can be derived from viscoelastic moduli, and this can be related to shear viscosity using the Cox-Merz rule.

This rule assumes that, for a rheological simple system such as a solution of linear polymers:

• Angular frequency (ω) in rads^-1 is analogous to shear rate (γ*) in s^-1
• Complex viscosity (η*) in mPas (or cP) is analogous to shear viscosity (η)

This rule is only truly applicable for these rheologically simple systems, and differences between complex viscosity and standard shear viscosity measurements increase with sample complexity, such as the presence of a dispersed phase. As many samples measured using DLS microrheology are rheologically simple then the Cox-Merz relationship is often valid. Analysis of a flow curve can then highlight key criterion for describing the viscosity-angular frequency dependence, particularly for non-Newtonian fluids. These include the following parameters which can be extracted using a suitable model fit (see microrheology utilities)

• Zero shear viscosity
• Shear thinning index
• Infinite shear viscosity

Figure 9: Complex viscosity (η*) versus frequency

1.4.5 Creep compliance (J)

The MSD data is also related to creep compliance (J) of the sample - which is an alternative representation of sample viscoelasticity and is a measure of how far the particle moves for a given applied force, the units being in Pa^-1. This can be calculated directly from the MSD and is displayed in the time domain as opposed to the frequency domain for G as shown in Figure 10.

Figure 10: Creep compliance (J) vs time

1.5 Microrheology utilities

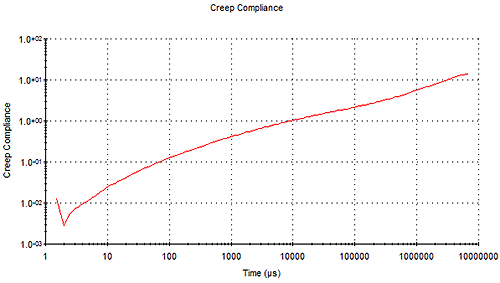
The Zetasizer software has a utilities section for the microrheology suite, which can be accessed via the Tools menu (Figure 11) or from the context menu (right click on a microrheology measurement).

Figure 11: Accessing the Microrheology Utilities panel

The Microrheology Utilities panel shows three charts - the Mean Square Displacement, the Viscoelastic moduli and the Complex viscosity.

On each page, rheological models are available that can be fitted to the data e.g. Figure 12 shows complex viscosity data that has been analysed using a Cross model.

It is important to note that not all models will be applicable to all datasets. In order to select appropriate areas of data only for subsequent model fitting (i.e. to remove excessively noisy data that can occur at the very shortest timescales), right click and drag the range pointers (the red triangles on the x axis) to the appropriate points on the chart.

Figure 12: Model fitting using the Microrheology Utilities

1.6 Exporting Microrheology data

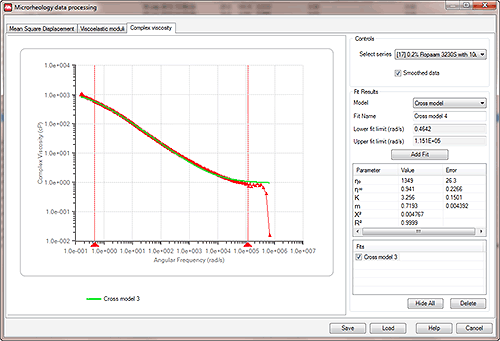
The microrheology results can be exported either from the File menu (shown in Figure 13) or from the Microrheology Utilities section using the save button. This latter approach is preferred and exports data in a format that can be easily imported in to the rSpace software for further analysis.

The Microrheology Utilities have two file options - save as .xml or save as .csv.

The parameters that are exported are as follows:

1.7 Importing Microrheology data in to rSpace

Microrheology data generated on the Zetasizer can be imported into the Kinexus rSpace software using the ‘Microrheology Import Sequence’ available in the rSpace software. This allows data to be combined or compared with rotational rheometry data and further analysis performed. The ‘Microrheology Import Sequence’ can be located using the rFinder search engine by entering the search term “microrheology” as shown in Figure 14. Clicking on the import sequence will prompt the user to select an appropriate csv file and the data will then be imported and displayed in both tabular and graphical form.

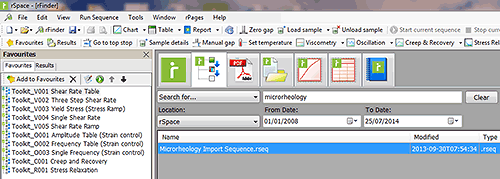
Figure 14: ‘Microrheology Import Sequence’ located using the rFinder search engine